Abstract
BACKGROUND: The present study aimed to validate a recently proposed algorithm for assistance titration during proportional assist ventilation with load-adjustable gain factors, based on a noninvasive estimation of maximum inspiratory pressure (peak Pmus) and inspiratory effort (pressure-time product [PTP] peak Pmus).
METHODS: Retrospective analysis of the recordings obtained from 26 subjects ventilated on proportional assist ventilation with load-adjustable gain factors under different conditions, each considered as an experimental case. The estimated inspiratory output (peak Pmus) and effort (PTP-peak Pmus) were compared with the actual-determined by the measurement of transdiaphragmatic pressure- and the derived PTP. Validation of the algorithm was performed by assessing the accuracy of peak Pmus in predicting the actual inspiratory muscle effort and indicating the appropriate level of assist.
RESULTS: In the 63 experimental cases analyzed, a limited agreement was observed between the estimated and the actual inspiratory muscle pressure (−11 to 10 cm H2O) and effort (−82 to 125 cm H2O × s/min). The sensitivity and specificity of peak Pmus to predict the range of the actual inspiratory effort was 81.2% and 58.1%, respectively. In 49% of experimental cases, the level of assist indicated by the algorithm differed from that indicated by the transdiaphragmatic pressure and PTP.
CONCLUSIONS: The proposed algorithm had limited accuracy in estimating inspiratory muscle effort and with indicating the appropriate level of assist.
Introduction
Proportional assist ventilation (PAV) with load-adjustable gain factors (PAV+) is a patient effort-driven mode of assisted ventilation, in which the ventilator provides pressure proportional to the patient's instant flow and volume, and thus is proportional to the elastic and resistive work load.1–3 Previous studies demonstrated the effectiveness of PAV+ relative to conventional assisted modes of mechanical ventilation.4–8 However, clinical use of this mode is limited, likely due to the lack of established criteria for titrating the level of support. The conventional method of assistance titration, based on tidal volume (VT) and breathing frequency may be hindered during ventilation on PAV+; with this mode, significant variability in VT has been observed, and patients retain their desired breathing pattern.4,9
A clinical algorithm has been introduced to titrate the level of assistance during ventilation on PAV+, which uses a noninvasive estimation of respiratory muscle output (maximum inspiratory pressure [peak Pmus]) and effort (pressure-time product [PTP] of peak Pmus.10 The level of support used peak Pmus to target a predefined range (5–10 cm H2O), which is estimated to correspond to the generally accepted range of inspiratory muscle effort (PTP of 50–150 cm H2O × s/min).
Clinical applicability of this algorithm was evaluated in a group of subjects on mechanical ventilation with various forms of acute respiratory failure.10 By adjusting the gain in PAV+ by using this algorithm, the majority of the subjects were successfully weaned from the ventilator. The investigators concluded that peak Pmus and the derived pressure time product of peak Pmus might serve as accurate surrogates of pressure generated by the respiratory muscles and inspiratory muscle effort, respectively.10 However, the calculation of peak Pmus and PTP-peak Pmus was based on assumptions that might result in significant discrepancies between estimated and actual inspiratory muscle output and effort, as measured by transdiaphragmatic pressure (Pdi) and the derived PTP-Pdi.
Su et al11 previously investigated the accuracy of this method in subjects who were critically ill. Peak Pmus and PTP-peak Pmus were compared with peak muscle pressure and effort calculated from esophageal pressure (Pes) at different levels of ventilator assist. Weak correlations were observed between both peak Pmus and peak muscle Pes, and the derived PTPs. However, this study was limited by the small number of subjects, the lack of gastric pressure measurement, and the lack of Pdi measurement. Furthermore, the investigators did not evaluate the accuracy of peak Pmus for predicting inspiratory effort and indicating the appropriate level of assist. The present study aimed to evaluate the association between estimated and the actual values of inspiratory muscle output and effort by comparing peak Pmus with Pdi, and PTP-peak Pmus with PTP-Pdi; identify confounding physiologic factors that contribute to discrepancies between compared variables; and validate the accuracy of the proposed algorithm for assistance titration.
QUICK LOOK
Current knowledge
During proportional assist ventilation with load-adjustable gain factors (PAV+), the conventional method of assistance titration, based on tidal volume and breathing frequency, may be hindered because significant variability in tidal volume has been observed in this mode and patients retain their desired breathing largely independent of mechanical load and assistance level.
What this paper contributes to our knowledge
The present study retrospectively evaluated an algorithm for assistance titration during ventilation on PAV+. The algorithm showed limited accuracy for indicating the appropriate assist level.
Methods
We retrospectively analyzed the recordings of 26 subjects who participated in 3 previous research protocols. The ethics committee of the hospital approved the study design, and informed consent was obtained from the subjects or their families. This study was performed at the Department of Intensive Care Medicine, University Hospital of Heraklion, Heraklion, Crete, Greece. All the subjects were ventilated on PAV+ (Puritan-Bennett 840 ventilator, Medtronic, PLC, Ireland) at different levels of assist, and instrumented with esophageal and gastric balloons. As part of the individual research protocols, 15 subjects were studied with and without an artificial increase in the elastic work of breathing, accomplished by applying sandbags to the entire surface of the chest and abdominal wall. Each subject at each experimental condition was regarded as an individual experimental case.
Measurements
Flow, volume, airway pressure (Paw), Pes, gastric pressure, and Pdi (Pdi = gastric pressure − Pes) pressures were measured on a breath-by-breath basis, as previously described.4,12 The proper position of the esophageal and gastric balloons was initially verified by using standard tests and procedures.13
Data Analysis
In each experimental case, at least 10 breaths over a period of 3 min were randomly analyzed and averaged to obtain breath variables for the corresponding experimental case. Breaths with a low-quality Pdi signal were excluded. Pdi was defined as the highest value of Pdi during inspiration, and the inspiratory effort per breath (PTP-Pdi) and PTP-Pdi/min were quantified.4,12 As previously described, the following were measured in each selected breath: the neural and mechanical inspiratory times; the difference between neural and mechanical inspiratory times (Δt); the rate of the rise of Pdi (dp/dt); the triggering delay; the intrinsic PEEP (PEEPi); and the presence of expiratory muscle activity and contribution of the diaphragm and inspiratory rib cage muscles to inspiratory output (see the supplementary materials at http://www.rcjournal.com).4,12,14 The estimated peak Pmus and the estimated inspiratory effort (PTP-peak Pmus) were calculated by using the formulas proposed by Carteaux et al10 as follow:
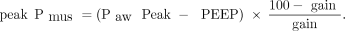
Where PawPeak = peak inspiratory Paw and gain = the level of assist.
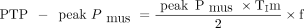
where TIm = mechanical inspiratory time and f = breathing frequency (see the supplementary materials at http://www.rcjournal.com).10
The differences were calculated between peak Pmus and Pdi (dP), and between PTP-peak Pmus/min and PTP-Pdi/min (ΔPTP); dP and ΔPTP/min were also expressed as the percentage of Pdi (dP%Pdi) and PTP-Pdi/min (ΔPTP/min% PTP-Pdi/min), respectively.
Correlations between peak Pmus, PTP-peak Pmus, dP, and ΔPTP with each of the following possible confounding physiologic factors were evaluated (as independent variables): dp/dt (an index of respiratory drive); PEEPi; presence of expiratory muscle activity (yes/no); ratio of gastric pressure to Pes changes during inspiration, an index of contribution of the diaphragm and inspiratory rib cage muscles to the inspiratory output); triggering delay; and difference between the mechanical inspiratory time and the neural inspiratory time (Δt).
Validation of the Proposed Algorithm
The validation of the proposed algorithm was performed by assessing the accuracy of peak Pmus to correctly classify the actual inspiratory muscle effort, determined by the PTP-Pdi. Specifically, in each experimental case, we assessed whether the measured PTP-Pdi was within the range of that predicted by the peak Pmus inspiratory muscle effort (ie, <50 cm H2O × s/min to peak Pmus of <5 m H2O 50–150 cm H2O × s/min to peak Pmus of 5–10 cm H2O and >150 cm H2O × s/min, to peak Pmus of >10 cm H2O, respectively). Analysis was performed in all experimental cases combined and in 3 subgroups, determined by the peak Pmus value: <5, 5–10, and >10 cm H2O (see the supplementary materials at http://www.rcjournal.com).
Statistical Analysis
Continuous variables are reported as the mean ± SD for normally distributed data, and median and interquartile range (IQR) for non-normally distributed data. Continuous variables were compared (2-tailed) by using the Wilcoxon test (paired sample). Linear regression analysis was used, and the coefficient of determination was calculated to examine the relationship between continuous variables. Analysis of residuals confirmed the assumptions of linearity. The agreement (bias) between variables was expressed as the mean of the corresponding differences. The limits of agreements were expressed as the mean ±1.96 SD, and 95% CIs of the bias were calculated by using the Bland-Altman method. The correlation between continuous variables was assessed by using Spearman's rho, followed (when indicated) by multiple regression analysis. Validation of the algorithm was performed by using receiver operating characteristic curve analysis. All statistical tests were 2-tailed, and P < .05 was considered to be statistically significant. Statistical analysis was performed by using MedCalc Statistical Software version 15.8 (MedCalc Software bvba, Ostend, Belgium). Statistical analysis was reviewed by an external statistician to confirm that no conflict existed between identified correlations and investigators' interpretations. The sample size required obtaining a size effect of 0.8 between compared variables for a 2-sided α of 0.05, and study power of 80% was calculated to be 25 cases.
Results
The recordings of 26 subjects with respiratory failure from different causes were included in the analysis. Subject demographic and clinical characteristics are presented in Supplementary Table 1 (see the supplementary materials at http://www.rcjournal.com). In 10 subjects, as part of the original study design, we retrieved recordings at different levels of ventilator assist (up to 4). Fifteen subjects were studied before and after the experimental increase in elastic respiratory work load, at either the same (10 subjects) or different (5 subjects) levels of assistance. Sixty-three different levels of assistance were identified, and a total of 725 sufficient breaths were available for analysis. The mean ± SD level of assistance was 50 ± 14.5%. Physiologic variables and breath characteristics (median values and IQR) are shown in Table 1.
Inspiratory Effort Indices and Breath Characteristics
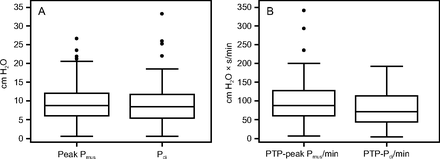
The median (IQR) difference between PTP-peak Pmus/min and PTP-Pdi/min was 14.65 (−13.52 to 45.615) cm H2O × s/min; the median (IQR) difference between peak Pmus and Pdi was 0.68 (−3.29 to 2.11) cm H2O. The PTP-peak Pmus/min (median, 87.15 cm H2O × s/min) was significantly higher than PTP-Pdi/per min (median, 71.19 cm H2O × s/min; P = .04). However, in 38 experimental cases (60.31%), the difference between the 2 variables was negative (PTP-peak Pmus was lower than PTP-Pdi/min). No significant difference was found between peak Pmus (median, 8.77) cm H2O and Pdi (median, 8.50; P = .45) (Fig. 1).
Box and whiskers plots, representing a comparison of Peak Pmus and Pdi (A), and PTP-Peak Pmus and PTP-Pdi (B).The lower and upper edges of each box represent the 25th and 75th percentiles, respectively. Median values are shown by the lines within each box. Whiskers represent adjacent values. Points denote outliers. Peak Pmus = calculated peak inspiratory pressure; Pdi = transdiaphragmatic pressure, PTP-peak Pmus = calculated pressure-time product of peak Pmus; PTP-Pdi = pressure-time product of Pdi.
A significant linear relationship was present between peak Pmus and Pdi (coefficient of determination, R2 = 0.346, slope = 0.5253, P < .001), and between PTP-peak Pmus and PTP-Pdi (R2 = 0.33, slope = 0.729, P < .001); however, there was significant scatter in the measurements. Scatter plots and corresponding regression equations are shown in Supplementary Figure 1 (see the supplementary materials at http://www.rcjournal.com).
Bland-Altman analysis revealed limited agreement between peak Pmus and Pdi, and between PTP-peak Pmus and PTP-Pdi (Fig. 2). The mean difference (bias), limits of agreement, and corresponding 95% CIs of bias are shown in Table 2. Correlations between peak Pmus, PTP-peak Pmus, the difference between peak Pmus and Pdi, and the difference between PTP peak Pmus and PTPPdi with each of the possible confounding physiologic factors are shown in Supplementary Table 3 (see the supplementary materials at http://www.rcjournal.com). Significant positive correlations were found between the rate of increase in dp/dt and both peak Pmus (rs = 0.49, P < .001) and PTP-peak Pmus (rs = 0.24, P = .03). The difference between peak Pmus and Pdi (dP) was inversely correlated with dp/dt ([Spearman's rank correlation coefficient] rs = −0.39, P = .001), which indicated an increase in dP with a decrease in dp/dt. A significant positive correlation was found between ΔPTP and the difference between mechanical and neural inspiratory time (rs = 0.28, P = .04).
Bland-Altman analysis presenting the mean difference (solid middle line) and 95% CI of the differences (±1.96 SD of the mean; dotted lines) between Pdi and peak Pmus (A) and between PTP-Pdi and PTP peak Pmus (B). Peak Pmus = calculated peak inspiratory pressure; Pdi = transdiaphragmatic pressure; PTPPdi/min =pressure-time product of Pdi per minute; PTP-peak Pmus/min = calculated pressure time product of peak Pmus per minute.
Mean Difference (bias) of peak Pmus-Pdi and PTP-peak Pmus-PTP-Pdi, Limits of Agreement (±1.96 SD of the mean), and 95% CIs for the Mean and for the Upper and Lower Limits of Agreement
Validation of the Proposed Algorithm
Based on the proposed algorithm at peak Pmus of <5, 5–10, and >10 cm H2O, inspiratory muscle effort was estimated to be <50, 50–150, and >150 cm H2O × s/min, respectively; accordingly, the level of assistance was proposed as excessive (overassist), adequate, or insufficient (underassist).
Overall, in 31 of 63 experimental cases (49.21%), the inspiratory effort determined by the PTP-Pdi was classified in a different range than that predicted by the calculated peak Pmus. The sensitivity and specificity of peak Pmus to predict the actual inspiratory effort and thus to correctly characterize the level of assist were 81.2% and 58.1%, respectively. The area under the receiver operating characteristic curve was 0.70 (95% CI 0.57–0.83; P = .012) (Fig. 3).
Receiver operating curve for the prediction of the actual range of inspiratory effort by the calculated peak inspiratory pressure (peak Pmus), showing an optimal criterion of <9.85 cm H2O.
Subgroup 1: peak Pmus <5 cm H2O
Peak Pmus was <5 cm H2O in 11 of 63 experimental cases, which suggests low inspiratory effort and an excessive level of assist. Inspiratory effort measured by PTP-Pdi/min was within the range predicted by peak Pmus in 7 of 11 experimental cases (63.64%). In the remaining experimental cases (4/11 [36.36%]), PTP-Pdi/min was within acceptable limits (50–150 cm H2O × s/min), which suggested an adequate level of assist.
Subgroup 2: peak Pmus of 5–10 cm H2O
Peak Pmus ranged between 5 and 10 cm H2O in 29 of 63 experimental cases, which indicated an adequate level of assist. Inspiratory effort measured by the PTP-Pdi/min was within the range of inspiratory effort predicted by peak Pmus in 18 of 29 experimental cases (62.07%). In 11 of 29 experimental cases (37.93%), PTP-Pdi/min was either <50 (31.03%) or >150 H2O × s/min (6.89%), which indicated excessive or insufficient ventilator assist, respectively.
Subgroup 3: peak Pmus >10 cm H2O
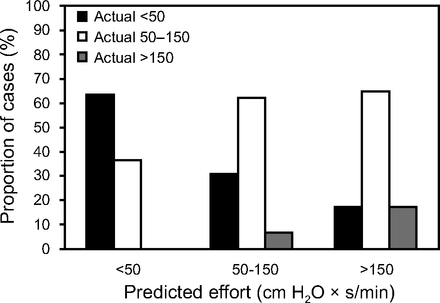
Peak Pmus was >10 cm H2O in 23 of 63 experimental cases, which indicated high inspiratory effort and insufficient ventilator assist. PTP-Pdi/min was within the range predicted by peak Pmus in 4 of 23 experimental cases (17.39%). PTP-Pdi/min ranged from 50 to 150 cm H2O × s/min in 15 of 22 experimental cases (65.21%), and was <50 cm H2O × s/min in 4 of 22 experimental cases (17.39%), which indicated adequate or excessive ventilator assist, respectively (Fig. 4).
Percentage of experimental cases with actual inspiratory effort (PTP-Pdi) of <50, 50–150, or >150 cm H2O × s/min, at each different range of predicted by peak Pmus inspiratory effort: <50, 50–150, and >150 cm H2O × s/min. Peak Pmus = calculated peak inspiratory pressure, PTP-Pdi: pressure-time product of transdiaphragmatic pressure per minute.
Discussion
The most significant findings of the present study were the following: (1) there was limited agreement between formula-derived estimates of peak Pmus and effort PTP-peak Pmus, and actual inspiratory pressure and effort measured by Pdi and PTP-Pdi, respectively, and (2) setting the ventilator assistance by using the proposed algorithm could result in either under- or overassistance in approximately half of the study cases.
With conventional modes of assisted ventilation, the assistance level is primarily determined based on the patient's breathing pattern; this frequently results in considerable dissociation between patient demands and ventilator output.15–19 Conversely, VT and breathing frequency may be considerably modified by ventilator settings.20 During ventilation on PAV+, titrating the assistance level by VT and breathing frequency may be of limited effectiveness. Significant variability in VT has been observed, and patients retain their desired breathing largely independent of mechanical load and assistance level.1,4,12
To simplify and unify titration of assistance level during ventilation on PAV+, Carteaux et al10 introduced a simple algorithm, as described earlier in this article. However, that study did not validate the estimated variables or the proposed algorithm for assistance titration. In the present study, we validated the proposed algorithm by evaluating the accuracy of peak Pmus to predict the actual inspiratory effort, as determined by the accepted standard method of PTP-Pdi. In up to 49% of cases, PTP-Pdi was in a different range from that predicted by peak Pmus. Consequently, in nearly half of the experimental cases, gain adjustment by using the proposed algorithm could result in either over– or under–ventilation assistance. The lowest accuracy of peak Pmus was present in the subgroup of experimental cases characterized by peak Pmus of > 10 cm H2O; in that subgroup, peak Pmus failed to predict the actual inspiratory effort in up to 83% of experimental cases.
Numerous studies have shown the deleterious effects of inappropriate assistance level (either excessive or insufficient) on the respiratory muscles. An excessive level of assistance results in diaphragmatic atrophy and contractile dysfunction.21–25 Mechanical ventilation–induced diaphragm atrophy is associated with diaphragmatic dysfunction, which has been related to unfavorable clinical outcomes.26–28 Excessive assistance may induce respiratory alkalosis, which, in patients with a preexisting low respiratory drive (ie, metabolic alkalosis and/or sedation) may promote periodic apnea.29,30 Furthermore, in patients with obstructive lung diseases ventilated in assisted modes, excessive assistance may lead to high VT and dynamic hyperinflation, which leads to triggering delay and ineffective efforts, thus adversely affecting patient outcomes.15,16 Conversely, when ventilator support is insufficient for patient demands, vigorous inspiratory efforts may result in self-inflicted lung injury.31–34 Furthermore, mismatch between ventilation demands and ventilator assistance may be associated with patient discomfort, increased work of breathing, and prolonged mechanical ventilation and ICU stay. The current study showed that the limited prediction value of peak Pmus was attributed to the disagreement between the estimated and actual inspiratory muscle output and effort.
Comparison Between peak Pmus and Pdi
Although the difference was not statistically significant, we found low agreement between the 2 variables, indicated by the broad limit of agreement in the Bland-Altman analysis, significant scatter of the measurements, and low coefficient of correlation in the regression analysis. The discrepancy between these variables could arise from either a misleading calculation of peak Pmus and/or different physiologic factors related to both ventilator and subject characteristics.
The proposed equation for peak Pmus does not include PEEPi; therefore, peak Pmus is expected to be underestimated in patients who exhibit PEEPi. The extent of pressure underestimation depends on the levels of assistance and PEEPi; at low assistance and high levels of PEEPi, underestimation increases. In addition, because pressure delivery in PAV is driven by patient effort, the presence of PEEPi reduces the fraction of the patient's effort that is being assisted, which leads to underestimation of the proportion of assistance being provided. However, the presence of PEEPi may contribute minimally to disagreement between these compared variables; although we included a relatively high proportion of subjects with COPD, we found a low level of PEEPi (median value of 1.5 cm H2O). At this level of PEEPi, underestimation is minimal, even at low levels of assist. Both ventilator and patient characteristics, including triggering delay, inspiratory muscle output, dynamic hyperinflation, expiratory and accessory muscle activity, and patient respiratory drive may also contribute (either separately or collectively) to the dissociation between peak Pmus and Pdi.
Collectively, for all experimental cases, respiratory drive was the sole factor that significantly correlated with the difference between the estimated and the actual peak muscle pressure; as respiratory drive increased, the difference in peak muscle pressure decreased. This association is likely attributed to associated changes in peak Pmus because we found a significant positive correlation between peak Pmus and the respiratory drive. Changes in respiratory drive may alter ventilator output, mainly through changes in triggering delay.18,35,36 Because the Paw value is the primary determinant in the peak Pmus calculation, any increase or decrease of Paw results in changes in the calculated peak pressure. Nevertheless, our data indicated that, for individual experimental cases, the difference between peak Pmus and Pdi should be attributed to multiple factors rather than strictly to changes in respiratory drive. For example, we found that peak Pmus was significantly higher than Pdi in experimental cases with relatively low respiratory muscle output and respiratory drive, and relatively high triggering delay. The inverse relationship was also observed.
Comparison Between PTP-peak Pmus and PTP-Pdi
We found a significant difference between the inspiratory effort estimated by the proposed formula and actual inspiratory effort measured by Pdi (PTP-Pdi). This finding was demonstrated by both the low agreement between compared variables in Bland-Altman analysis as well as regression analysis. Disagreement between PTP-peak Pmus and PTP-Pdi can mainly be attributed to the assumptions on which the calculations were based. First, PTP-peak Pmus was calculated as the area under the corresponding waveform during the inspiratory time when assuming that the rate of increase of inspiratory muscle pressure is constant (linear during neural inspiration); this resulted in a triangular area under the waveform. Nevertheless, results of physiologic studies indicate that the rate of increase in inspiratory muscle pressure (Pmus) or Pdi typically exhibits a concave or convex shape.37 Consequently, the area under the Pdi waveform or pressure generated by respiratory muscles, or is expected to either be lower (in a concave shape) or higher (in a convex shape) compared with the area in the linear waveform (Supplementary Fig. 2 [see the supplementary materials at http://www.rcjournal.com]).
Second, PTP-peak Pmus was calculated based on the assumption that mechanical and neural inspiratory times were equal. Ideally, during assisted modes, the neural time may coincide with mechanical time. However, mechanical inspiration typically ends either before or after the end of neural inspiration,17,18,35,38 even in modes in which inspiratory effort drives ventilation, as in PAV/PAV+.4,12 Because PTP-peak Pmus is calculated by using the mechanical inspiratory time for a specific peak Pmus value, the derived PTP is expected to vary with the mechanical time (relative to neural time). In nearly all experimental cases in the present study, the mechanical time was higher than neural time, largely due to triggering delay.
The contribution of the difference between the neural and mechanical inspiratory time to the difference between PTP-peak Pmus and PTP-Pdi was supported by a significant positive correlation between ΔPTP and the difference between neural and mechanical inspiratory times; increased time difference was associated with an increased difference between PTP-peak Pmus and PTP-Pdi. Evidently, in individual experimental cases, the difference between PTP-peak Pmus and PTP-Pdi can be attributed to by a combination of the above, at variable degrees of participation. Our findings were in agreement with those of a study by Su et al.11 peak Pmus and the derived PTP were compared with Pmus, as calculated from Pes. Although the design and study population varied between the 2 studies, both revealed a weak correlation between estimated and actual inspiratory muscle output and effort.
Limitations and Clinical Implications
This study was a retrospective validation of proposed formulas to estimate peak muscle pressure and effort during ventilation with PAV+ and not clinically evaluate the proposed algorithm. The number of patients included here was lower than that in the study that proposed the algorithm. Analysis of our data showed high variability in the causes of error in the estimated values, which suggested that other sources of error may be identified by using a larger patient sample. The aim of setting the level of assist based on patient effort to avoid over- or underassist remains undisputed. However, this study highlighted the complexities of accurately estimating patient effort without invasive measurements and emphasized the need for further research in this direction. When adjusting the level of assist in PAV+, the caregiver may use the proposed algorithm as a starting point and may then adjust the assist level according to patient comfort and gas exchange.
Conclusions
This study showed that, in subjects on mechanical ventilation and with the PAV+ mode, there was significant disagreement between the actual and estimated respiratory muscle pressure and effort due to factors related to both subject and ventilator characteristics. Estimated peak inspiratory pressure showed limited accuracy in predicting actual inspiratory muscle effort; therefore, in nearly half of the analyzed experimental cases, adjusting the assistance level with the proposed algorithm could have led to over– or under–ventilator assist.
Footnotes
- Correspondence: Eumorfia Kondili MD PhD, Department of Intensive Care Medicine, University Hospital of Heraklion, Voutes 71110, Heraklion, Crete, Greece. E-mail: kondylie{at}uoc.gr.
Drs Amargiannitakis and Gialamas contributed equally to this work.
Drs Kondili, Vaporidi, and Georgopoulos have received lecture fees (honoraria) from Covidien.
Covidien was not involved in any aspect of the design or conduct of the study, the data analysis, or the manuscript preparation and presentation.
Supplementary material related to this paper is available at http://www.rcjournal.com.
- Copyright © 2020 by Daedalus Enterprises