High-frequency ventilation got its start in the United States from a paper by Dr Mirosolav Klain, an anesthesiologist who described a high-frequency jet ventilator (HFJV)1 he built using a fluidic control circuit purchased as a demonstration kit from Corning Glass Works.2 Based on his description of the circuit, we built an HFJV and humidification system that we used to ventilate neonates clinically for over 10 years.3–5 In those days, high-frequency ventilation was primarily the interest of neonatologists. A review of the high-frequency ventilation “state-of-the-art” at the time was published in this journal in 1984.6 Neonatologists conducted the first large randomized trial of high-frequency ventilation in premature infants in 1989.7 For that trial, high-frequency oscillatory ventilation (HFOV) was used because of concerns with necrotizing tracheobronchitis reported from use of improper humidification during HFJV. The Gould high-frequency ventilator was invented during this time, later to become the Sensormedics 3100 high-frequency oscillator. That ventilator remains in use today, essentially unchanged (except for a larger drive motor for use with adults). The only other high-frequency ventilator available in the United States is the Bunnell Life Pulse HFJV, designed for neonates.
Amazingly, after over 30 years of countless research studies, it was only in 2010 that a meta-analysis finally supported the hypothesis that HFOV is superior to conventional mechanical ventilation.8 In fact, we believe that was the only instance in history of a study concluding that one particular “mode” of ventilation had a survival benefit compared to other modes. And even more surprising, despite all the studies of the technology of HFOV over 3 decades, Bostick et al9 are the first to provide evidence of carbon dioxide entrainment during HFOV. Their study showed that certain combinations of pressure amplitude and mean airway pressure can result in negative airway pressures that cause a retrograde CO2 entrainment (ie, into the inspiratory limb of the patient circuit), increasing the effective dead space and possibly contributing to the development or persistence of hypercapnia.
While the study by Bostick et al9 was elegantly designed and their results convincing, their analysis of the results provided little basis for understanding why retrograde CO2 entrainment occurs. In the Discussion these authors comment that this phenomenon is complex and has not been fully explored. What follows is a simple model and circuit analysis that provides theoretical support for the Bostick et al9 study observations.
Model Creation
To put the authors' intent and results into the proper perspective, we need to start with a simple model of the HFOV and lung simulator system and apply some very basic circuit analysis. Figure 1 shows the electronic circuit analog that represents this system.
An electrical circuit analog of a high-frequency oscillatory ventilator connected to a patient. See text for definitions of terms.
Simplifying Assumptions
1. Because the flow to the CO2 monitor (V̇sample) and from the CO2 source (V̇CO2) are very small, compared to the other flows, they can be ignored.
2. The flow from the ventilator can be approximated by a sine function. This is justified by the Fourier theorem stating that any periodic waveform can be represented by the sum of a set of sinusoidal waveforms. This model can then be described by a system of equations:
V̇osc(t) = oscillatory flow from the ventilator diaphragm as a function of time, t
V̇tot(t) = total flow from oscillator and bias flow as a function of time
V̇valve = flow through the exhalation valve
V̇bias = bias flow to generate mean airway pressure
V̇leak = flow through the leak around the endotracheal tube
V̇lung = flow into the lung
V̇sample = flow to the CO2 monitor
V̇CO2 = flow from the CO2 source simulating the body's carbon dioxide excretion
Paw(t) = airway pressure (ie, transrespiratory system pressure difference) as a function of time, t
Rleak = resistance of the path of the leak flow
Rtube = resistance of the endotracheal tube
Rlung = resistance of the lungs
Rvalve = resistance of the exhalation valve
Rsample = resistance of the CO2 monitor sample path
Clung = compliance of the lungs
The flow from the oscillatory diaphragm is modeled as a sine function as follows:
where
V̇osc(t) = oscillatory flow from the ventilator diaphragm as a function of time, t
ΔV̇ = flow amplitude relative to mean flow (V̇bias)
sin = sinusoidal function of time
π = pi ≈ 3.14
f = frequency (Hz)
t = time (s)
Model Equations
The SensorMedics 3100 high-frequency oscillatory ventilator is a relatively simple device that does not use feedback control for directly setting either flow or pressure waveforms. Rather, the operator adjusts the power to the oscillating diaphragm, which then alters the flow amplitude, and the indirect result is the measured airway pressure amplitude (ΔP, peak or maximum pressure minus trough or minimum pressure for a cycle, sometimes called peak-to-peak amplitude). For a given power setting, the resultant pressure waveform is a function of the frequency, the impedance of the system, and the set percentage inspiratory time. The waveform will not be symmetrical about the mean airway pressure (like a sine wave) if the percentage inspiratory time is not set at 50%, but we will assume it is for the purpose of gross performance analysis.
Using the superposition theorem for electrical circuits, we can calculate each component of airway pressure, Paw(t), as the sum of the pressures due to V̇osc(t) and V̇bias.
where
Paw(t) = airway pressure as a function of time, t
Posc(t) = oscillating pressure as a function of time
P̄aw = mean airway pressure
Zsys = the magnitude of the impedance of the network of resistances and lung compliance
Because V̇osc(t) is sinusoidal, Posc(t) is also sinusoidal and may be expressed as:
where
ΔP = pressure amplitude setting on the SensorMedics 3100 ventilator. Note that the ΔP setting on the ventilator is a peak-to-trough amplitude. Thus, for a 50% inspiratory time setting this is approximately double the amplitude in Equation 3, because the amplitude of a sine wave is by convention relative to the mean value.
To calculate Zsys we first combine Rtube and Rlung and the capacitive reactance of the lungs. Capacitive reactance (XC = 1/2πfC) is a frequency dependent resistance to flow, which decreases as the frequency of the flow waveform increases. Because these components are in series, they are simply additive, and we will call the sum respiratory system impedance Zresp:
The resistances of the leak and the exhalation valve are in parallel, so their combined resistance is given by:
and thus
Rleak–valve and Zresp are in parallel, so their combined impedance is:
Note that as frequency increases, Zresp decreases (Equation 4) and thus Ztot decreases:
Under steady state conditions, flow into the lungs due to V̇bias decays to zero as lung pressure rises to the level of mean airway pressure. Mean airway pressure (P̄aw) is determined by the V̇bias, and the parallel resistance combination of the leak around the endotracheal tube cuff and the exhalation valve:
By conservation of mass we have an expression for the total flow through the system:
Using the superposition theorem, we can calculate each component of V̇tot as the sum of flow due to Posc(t) and P̄aw. Consider the situation if the oscillator frequency is set to zero. Then Posc(t) is zero and the only flow through the inspiratory limb is from V̇bias. The total flow is thus:
which indicates that V̇tot is constant (because P̄aw is constant) and always positive, so there can be no CO2 rebreathing (Fig. 2). On the other hand, if the oscillatory frequency is > 0 and V̇bias is turned off, then:
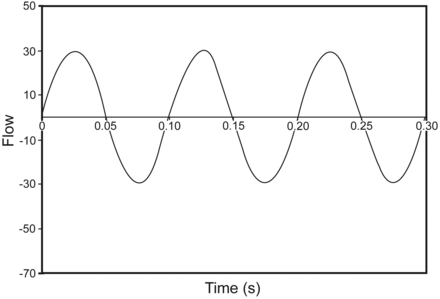
indicating that V̇tot oscillates and goes negative once per cycle, and there is always CO2 rebreathing (Fig. 3). With both oscillatory and bias flow active, the total flow is the sum of the 2 flows:
Graph of constant bias flow component of total flow during high-frequency oscillatory ventilation.
Graph of oscillatory flow component of total flow during high-frequency oscillatory ventilation.
Because V̇osc(t) is sinusoidal, it oscillates between +ΔV̇osc and –ΔV̇osc. Because ΔV̇osc is measured relative to V̇bias, if V̇bias is set equal to ΔV̇osc, then when V̇osc(t) reaches its lowest value, V̇tot will be zero, and retrograde flow of CO2 cannot occur (Fig. 4). If V̇bias falls below ΔV̇osc, then V̇osc(t) becomes negative and CO2 entrainment occurs. When V̇bias is larger, then V̇osc(t) is positive and entrainment is prevented.
Graph of total flow (bias flow plus oscillatory flow) during high-frequency oscillatory ventilation.
In Equation 13, if Rleak-valve happened to be the same as Ztot, then V̇osc(t) would be zero at its lowest value when mean airway pressure equaled the oscillatory pressure amplitude, ΔP, as shown in Figure 5. In this figure, pressure amplitude, ΔP, is defined relative to the mean value, as is the convention in mathematics. However, the ventilator measures ΔP as a peak-to-trough (also known as peak to peak) value. Thus, if the denominators in Equation 13 were equal, retrograde flow of CO2 would occur when ΔP was set equal to twice the P̄aw.
Graph of airway pressure (ie, transrespiratory system pressure difference) during high-frequency oscillatory ventilation. Note that the pressure amplitude displayed on the ventilator (ΔP) is a peak-to-trough amplitude, whereas the pressure amplitude of the electronic model (sine wave) is relative to the mean pressure. Therefore the model amplitude is equal to ΔP/2 for a symmetrical waveform (ie, inspiratory time setting = 50%).
Of course in reality, Ztot must always be higher than Rleak-valve, because it includes both Rleak-valve and Zlung. That means retrograde CO2 flow will occur at a ΔP value on the ventilator less than twice P̄aw. The higher the Ztot, the lower the ΔP threshold required to allow the phenomenon to occur.
Comparison of Model Results With Experimental Results
“Negative pressure was detectable within the inspiratory limb of the HFOV circuit and varied inversely with mean airway pressure.” Bostick et al9 have shown that during HFOV with typical settings, airway pressure does go below zero. Furthermore, as predicted by Equation 2, airway pressure becomes more negative as either ΔP increases or P̄aw decreases.
“CO2 was readily detectable within the inspiratory limb and was proportional to the negative pressure generated.” Retrograde CO2 flow does occur and is a function of the relative values of ΔP and P̄aw. As predicted by Equation 13, Figure 2 of the Bostick et al9 paper shows that airway pressure becomes zero (and retrograde flow of CO2 becomes possible) when ΔP is somewhat less than twice the value of P̄aw.
Removal of . . . cuff leak increased the amount of CO2 entrainment. . . . Retrograde CO2 entrainment was reduced with a higher bias flow . . . ” Consideration of Equation 9 offers some explanation of these results. If P̄aw depends on Rleak–valve and V̇bias, then eliminating the leak path (inflating the endotracheal tube cuff) will increase the resistance to V̇bias, because all bias flow must go through the exhalation valve. Now to maintain the same P̄aw we must either decrease V̇bias or adjust the exhalation valve so that it has lower resistance. If we decrease V̇bias, then it is more likely that it will be exceeded by the flow amplitude, and hence retrograde CO2 will occur (Equation 13). On the other hand, if we adjust the exhalation valve and maintain the same V̇bias, then retrograde CO2 is less likely to occur.
“CO2 entrainment was decreased as frequency increased.” This result is contrary to the prediction of Equation 13. For a simple model composed of a resistance and capacitive reactance in series, impedance decreases monotonically as frequency increases (Equation 4). Thus, increasing frequency should have decreased the denominator of the last term in Equation 13, increasing the amplitude of the oscillatory flow relative to the bias flow, and increasing CO2 entrainment. What this indicates is that the mathematical model is too simple to cope with frequency effects. Indeed, at high frequencies inertance plays a role. For example, for a series combination of resistance, capacitive reactance, and inductive reactance, total impedance is high at low frequencies, decreases to a minimum as frequency increases (the resonance frequency), and then increases to infinity. Apparently the mechanical and animal models used by Bostick et al9 had inertance, despite the fact that they only recorded resistance and compliance, and they were on the portion of the impedance curve where total system impedance increased with frequency. Inertance is difficult to model in this situation, because, unlike compliance, it is distributed throughout the system, and appropriate values for inertance in each branch of the model are unknown.
Bostick et al9 have provided evidence from a mechanical model and an animal model that during HFOV, CO2 may be entrained into the inspiratory limb of the patient circuit and thus add a “dead space” effect that may alter the overall level of ventilation. We have provided a simple electronic model and circuit analysis that explains this effect in terms of operator set ventilator variables such as pressure amplitude, mean airway pressure, ventilatory frequency, and bias flow. Understanding this model should help clinicians better predict the outcomes of various HFOV scenarios.
Footnotes
- Correspondence: Robert L Chatburn MHHS RRT-NPS FAARC, Respiratory Institute, M-56, The Cleveland Clinic, 9500 Euclid Avenue, Cleveland OH 44195. E-mail: chatbur{at}ccf.org.
Mr Chatburn has disclosed relationships with Alpha-1 Antitrypsin Foundation, Breathe Technologies, CareFusion, Covidien, Dräger, Hamilton, IngMar, Newport, Philips, Radiometer America, ResMed, Strategic Dynamics, and Teleflex. Dr El-Khatib has disclosed no conflicts of interest.
See the Original Study on Page 1865
- Copyright © 2012 by Daedalus Enterprises Inc.